Structural Vibration Solutions A/S
Structural Vibration Solutions A/S is the developer of the ARTeMIS Modal software for Operational Modal Analysis. We are focused on the mission to be the leading provider of Operational Modal Analysis solution in the world and to follow our vision to develop Structural Vibration Solutions that every engineer can use. Structural Vibration Solution A/S was founded 1st of March 1999 as a spin-off company from Aalborg University in Denmark. In 2009 we celebrate our 10 years anniversary with clients and users all around the globe.
Company details
Find locations served, office locations and our distributors
- Business Type:
- Software vendor
- Industry Type:
- Noise and Vibration
- Market Focus:
- Globally (various continents)
- Year Founded:
- 1999
About us
Structural Vibration Solutions A/S is the developer of the ARTeMIS Modal software for Operational Modal Analysis. We are focused on the mission to be the leading provider of Operational Modal Analysis solution in the world and to follow our vision to develop Structural Vibration Solutions that every engineer can use
The company was founded March 1, 1999 as a spin-off from Aalborg University in Denmark. Our patented software is today used e.g. by mechanical engineers for modal analysis of operating machinery and components, and by civil engineers for ambient vibration analysis of large structures like bridges and buildings.
Structural Vibration Solutions A/S is located at NOVI Science Park, which is one of northern Europe’s most respected science parks. The location plays an important role as NOVI Science Park secures the close relations between the company and the research from Aalborg University.
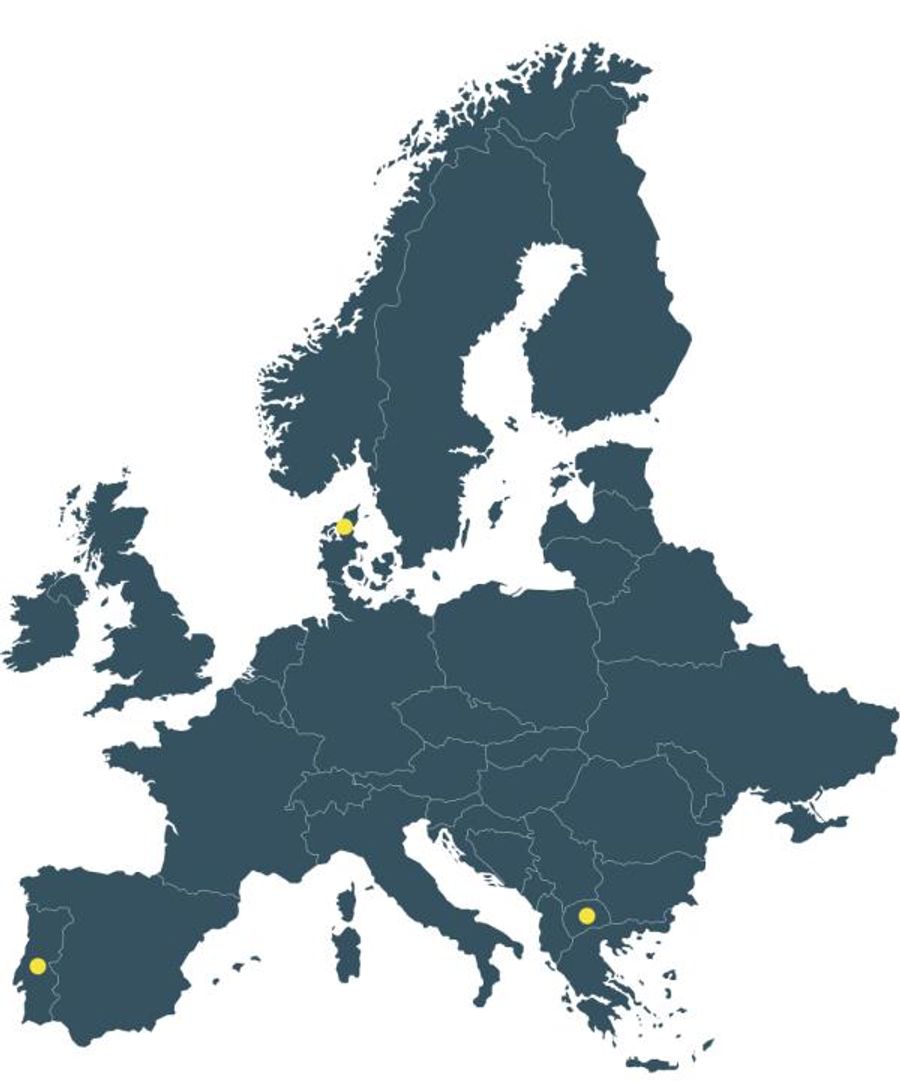
Our Departments
Today, SVS has departments in Denmark, Portugal and Republic of Macedonia. The software is developed in Denmark and Republic of Macedonia, and sales support for distributors as well as direct sales are handled from Portugal. The main office is located in Aalborg, Denmark at NOVI Science Park.